Home » Problems » Shock Wave: bvpT24

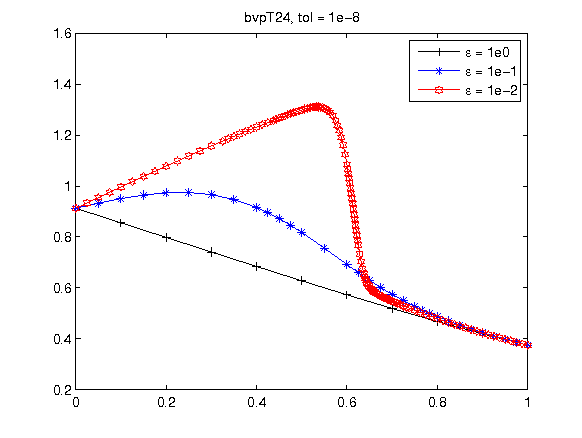
Shock Wave: bvpT24

 

 

 

shock wave problem: bvpT24
Contributor:  testset of J.R. Cash
Discipline:  fluid dynamics
Accession:  2013

 

 

 

Short description:

The problem describes a shock wave in a one dimension nozzle flow. The steady state Navier-Stokes equations generate a second order differential equations that is reduced to a first order system of 2 equations.

Applicable solvers:

all the solvers supported by the Test Set.

 

Plots of the solution <- click to generate the plots of the solution and the textual output

 

 

Mathematical description:

Consider a shock wave in a one dimension nozzle flow. The steady state Navier-Stokes equations give

    \begin{equation*} \begin{array}{ll} \lambda A(t) z z'' = \Big(\frac{\displaystyle{1 + \gamma}}{\displaystyle{2}} -\lambda A'(t)\Big) z z^{'} - \frac{\displaystyle{z'}}{\displaystyle{z}} - \frac{\displaystyle{A'(t)}}{\displaystyle{A(t)}} \Big(1 - \big(\frac{\displaystyle{\gamma - 1}}{\displaystyle{2}}\big) z^2 \Big) , &\\ A(t) = 1 + t^{2}, \;\;\; \gamma = 1.4, \;\;\;z(0) =0.9129, \;\;\; z(1) = 0.375 \\ \end{array} \end{equation*}

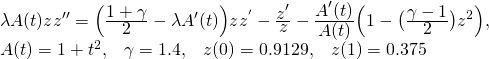
where t is the normalized downstream distance from the throat, z is the normalized velocity, A(t) is the area of the nozzle at t , with

    \[z \in \mathbb{R} , \;\;\; t\in [0,1]. \]

We write this problem in first order form by defining y_1=z and y_2=z', yielding a system of differential equations of the form

    \begin{equation*} \left(\begin{array}{c} y_1\\ y_2 \end{array}\right)'= \left(\begin{array}{c} y_2\\ \frac{1}{\lambda A(t) y_1}f(t,y_1,y_2) \end{array}\right), \end{equation*}

where

    \begin{equation*} f(t,z,z') = \Big(\frac{\displaystyle{1 + \gamma}}{\displaystyle{2}} -\lambda A'(t)\Big) z z^{'} - \frac{\displaystyle{z'}}{\displaystyle{z}} - \frac{\displaystyle{A'(t)}}{\displaystyle{A(t)}} \Big(1 - \big(\frac{\displaystyle{\gamma - 1}}{\displaystyle{2}}\big) z^2 \Big), \end{equation*}

with

    \[ (y_1,y_2)^T \in \mathbb{R}^{2} , \;\;\; t \in [0,1]. \]

The boundary conditions are obtained from

    \begin{equation*} \left( \begin{array}{cc} 1 & 0 \\ 0 & 0 \\ \end{array} \right) \left(\begin{array}{c} y_{1}(0)\\ y_{2}(0) \end{array}\right) + \left( \begin{array}{cc} 0 & 0 \\ 1 & 0 \\ \end{array} \right) \left(\begin{array}{c} y_{1}(1)\\ y_{2}(1) \end{array}\right)=\left(\begin{array}{c} 0.9129 \\ 0.375 \end{array}\right). \end{equation*}

Given its simple appearence, the BVP turns out to be a surprisingly difficult numerically. An O(\sqrt{\epsilon}) shock develops, whose location depends on \epsilon.

Singular-perturbation-type problems usually require a  continuation method to solve them .For this BVP, however, many  steps need to be taken.

 

Download: